1.
- You know this book by the physicist, A Short (- something or other -) About Time, said to be the most bought and not read book in human history? Have you read it?
- Didn't buy it but tried to read it in a bookstore.
- The physicist says, maybe someplace else, I'm not sure, that if we want to know how aliens will treat us when they land on our planet we should look at how those of us with high technology treat those with lower technology.
- They'll massacre most and enslave the rest.
- Yes.
- I guess people bought the book thinking that to compete they had to be up to date on technology and then didn't like reading the part about technology dooming the species to massacre each other and finally be massacred by aliens. Did you get far enough yourself to find out if he talked about societies that didn't organize themselves as parts in a machine?
- The first societies* where prestige of doing things well was tied to giving away the products of doing things well. Wealth wasn't accumulated, so wasn't inherited. And the technical knowledge responsible for accumulation, also not inherited, could not become the basis of social class.
- And not lead to massacring and enslaving each other.
- I am among those who couldn't finish the book, but I'm willing to bet it's not there.
- What about another bet? What are the chances alien invaders come from the end of the line of technological evolution rather than the beginning?
- Since the exceptions to technology and slavery appear only at the beginning of our history and in scattered small societies that have been isolated from history, isn't it likely aliens will travel the same path?
- We've had a lot more bad societies than good.
- Yes.
- And the only good societies we've had don't do well when contacted by the bad.
- They don't. The good don't survive.
- Will the bad societies survive?
- They have, so far.
- Suppose though slavery, massacre, inherited hierarchy destine us for destruction. On the other hand, the archaeological record and continuing survival of isolated communities show that good societies are stable and long lasting.
- So if good societies that go bad self-destruct, and good societies that stay good survive, and there is no necessity for good societies to go bad, then our reasoning is wrong: it is infinitely more likely we'll be contacted by people who stayed good, because there are more good societies than bad out there in space.
- As you said: if good societies don't all go bad. And if they get technology.
- What if technology always makes us bad and without technology we'll always be conquered by those with?
- Then aliens will come massacre and enslave us.
- Look on the bright side: every year that we continue to survive and the aliens don't come to get us is an argument that they don't want to. They have better things to do.
- And then like them we'll someday work out how to use technology and stay good.
- Do you know what else is encouraging?
- What?
- No one reads that guy's book.
Further Reading:
Einstein & Intellectual Physics
The Technology Of Good (And Other Stories)
Democracy & Inequality
____________________
* See The First Culture
2.
- Hierarchical technological societies destroy the simple sharing societies, and end up destroying themselves. If simple sharing societies got technology, reason taking the place of ritual, they'd be able to protect themselves from the hierarchical, technological societies. Assuming there is no beginning or end to time, and no beginning or end to space - we have already found thousands of planets orbiting other stars - since two types of society are destined for destruction and a third type not necessarily, the aliens that arrive one day to visit us will most like be from the third type, sharing and technological. You said technology may always make us bad. Could you go into that a little more?
- The Situationists* in the 50s and 60s came up with the brilliant idea to extend Marx's theory of alienation with Rousseau and Plato's description of society as imitation, representation, or in the Situationist's word, spectacle. When a worker is paid to make a product it is taken from him, and is no longer part of his own history, his own life story. Products in the world of the market, of employment, buying, and selling, are alien to the individual who made them, but acquire a mysterious social meaning for their buyers, possessing them becomes a symbol of their social role. The Situationists said that each of us in society works to make our social role, and were both alienated from our "self" - the product we've made of ourselves - and acquire for ourselves a mysterious symbolic value derived from the power and security that comes from having a place in society.
- What about private life?
- Trading for making money is without limit, and being without limit inconsistent with the defined goal-seeking nature of life. This according to Aristotle. He limited profit seeking to activities outside the home and only for the sake of securing the home.
- So he thought everyone in the market, producers, and buyers and sellers too, risked alienation if they didn't have home life. The Situationists said those times are over, because we ourselves are now the product, and we take that product self home with us. Is that right?
- Right. The Situationists wanted to make a revolution and counted on the ever increasing alienation from self and world to find them supporters. The '68 student revolt is tied directly to their agitation.
- So what happened?
- Do you think when we work to make a self in our society of spectacle, invent a role for ourselves, this process is like a worker making a product for an employer who takes it from him to sell?
- We don't have our self, after we make it, taken from us. We play it out.
- Yes. It is true that we intuitively know the difference between the made self and the self without artificial construction that is possible in life at home, but as we participate in the society of spectacle, playing our role, we feel safe and secure.
- Secure and alienated both. Not too promising for finding companions in revolution.**
- It may be difficult or impossible for a sharing technological society to develop out of hierarchical ones.
- Should we expect then a lower class of alien invaders?
- Not necessarily. We shouldn't expect hierarchical technological societies to go anywhere but to their destruction, in general.
- In general?
- We can't rely on history, development of a society as a whole, we can't say as did Marx and the Situationists that it contains the seeds of its own reform. However some individuals in the society likely do contain those seeds, and nothing in what we've said prevents them from planting them.
- And given an infinite amount of time and place for experiment, some are bound to survive.
3.
On Two New Sciences, Galileo, 1638:
Salviati: These difficulties arise because we with our finite mind discuss the infinite, attributing to the latter properties derived from the finite and limited. This, however, is not justifiable; for the attributes great, small, and equal are not applicable to the infinite, since one cannot speak of greater, smaller, or equal infinities. An example occurs to me which I shall refer to your consideration, Signor Simplicio, since it was you who started the discussion. I take it for granted that you know which numbers are squares and which are not.
Simplicio: I am aware of the fact that a square number arises through the multiplication of any number by itself; for example, 4 and 9 are square numbers formed from 2 and 3.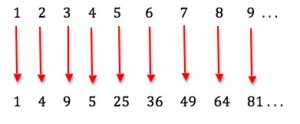
Salviati: Excellent. You remember also that just as the products are called squares, the factors, that is, the numbers which are multiplied by themselves, are called sides or roots. The remaining numbers, which are not formed from two equal factors, are called non-squares. If then I state that all numbers, squares and non-squares taken together, are more numerous than the squares taken alone, that is an obviously correct proposition, is it not?
Simplicio: It cannot be denied.
Salviati: If now I ask you how many squares are there, one can answer with truth, just as many as there are roots; for every square has a root, every root has a square, no square has more than one root, no root more than one square.
Simplicio: Entirely correct.
Salviati: Again, if I ask how many roots are there, one cannot deny that they are just as numerous as the complete number series, for there is no number which is not the root of some square. Admitting this, it follows that there are just as many squares as there are roots, since they are as numerous as the roots and every number is a root. Yet we said at the outset that all numbers are more numerous than all squares, since the majority of the former are non-squares. Indeed, the more numbers we take, the smaller is the proportion of squares ; for up to 100 there are 10 squares, that is, one tenth are squares ; up to 10000, one hundredth; up to 1000000, only one thousandth. Still up to an infinitely large number, granting we can conceive it, we were compelled to admit that there are just as many squares as numbers.
Simplicio: What is to be our conclusion?
Salviati: I see no escape except to say: the totality of numbers is infinite, the totality of squares is infinite, the totality of roots is infinite; the multitude of squares is not less than the multitude of numbers, neither is the latter the greater; and finally, the attributes equal, greater and less are not applicable to infinite, but solely to finite quantities.
- Your turn.
- I don't know. I'm expected to outdo Galileo?
- Yes. What do you have to say?
- The infinite is an idea, but not an idea about the world.
- What else can it be about?
- About both us and the world, about something we do in the world.
- What?
- Operate a machine of thinking. We take what we have and add one. Then take that and add one. We instruct ourselves to continue doing this. The infinite is a sort of recipe for action.
- A program.
- Yes. We can follow a recipe to construct an infinite series of odd numbers, like we can for all numbers. We imagine that the odd infinite must be smaller than the all number infinite because the all number series also includes the even numbers which also are infinite. Imagine we count at the rate of one unit per second.
- We operate the mental machine once per second.
- Yes. We don't see a larger or smaller infinite. We don't see a thing, "the infinite" at all. Ideas are collected experiences we see all together when we stop acting and rest. Infinites, continual action by recipe, cannot be ideas, cannot be seen.
- Then what are we doing when we talk about larger and smaller infinites?
- We imagine that the counting in our mind is shown in a movement in space. Each time we count one more we move a little forward. It looks like the set of all numbers is moving forward more than the set of odd or even numbers. When we get to 2 for all numbers, we have taken two steps, but for the even or odd numbers, only the first.
- We seem to be packing more movement and distance covered in the same infinite counting?
- Yes. Counting odd numbers and even numbers and squares is slower, covers less distances.
- So when we talk about bigger and smaller infinites we are really comparing speed of constructing infinite series.
- Right. Now this has some rather amazing implications for social life.
- Here we go.
- Social roles both provide security and are alienating. They provide security by giving us a sense of power, the power to do repeatedly what is done in our particular role. Social role is a kind of infinite. We imagine how we could "operate" our role on whatever the world throws at us, always adding one more instance of successful performance. On the other hand, social roles are alienating. We imagine that if we had no particular role at all, were instead all roles, we'd be like the set of all numbers not only odd, even, or squares, we'd be "larger infinities", we'd get further quicker, we'd cover more ground in life.
- This reminds me of the paradox, Zeno's arrow. In one second it will hit the target. In half a second it is half way there, in a quarter second more it gets closer, in an eighth of a second more, closer still, in a sixteenth of second more, closer still. We can operate this machine of adding ever smaller periods of time, and the arrow seems to never get to the target. Are you saying something similar?
- When we choose a social role, we are like the arrow traveled half way.
- I see that. Like odds or evens or squares.
- Imagine then we take on further specificity of social role. For example, Asian, female, Christian, homosexual student life, the subject of a movie I saw today. Each new role seems to be adding to life, but halves the ground covered, like odd numbers are half of whole numbers. The more specific the roles we take on, the smaller our infinite, and that makes us feel alienated. Our power is increasing but life is shrinking.
- Like the arrow, really we're getting nowhere.
*The Society Of Spectacle, Guy Debord.
See Debord's Film
** The few who aren't happy in their roles in society are happy in their roles as protestors against society: members of the Situationist group accuse each other of only playing at being a Situationist...